Research Areas
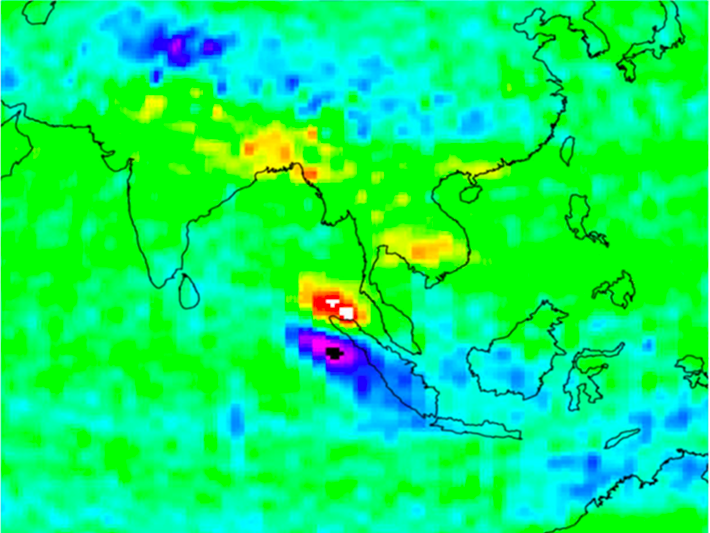
Earthquakes and GRACE
Since launch in 2002, data from the Gravity Recovery and Climate Experiment (GRACE) have provided a synoptic view of mass change associated seismic cycle and other processes. As part of the GRACE and now the GRACE-FO science teams, we have studied the long-wavelength gravity signal associated with varied earthquakes, including the great subduction zone earthquakes, as well as strike-slip and normal faulting events that also produce discernible gravity changes.
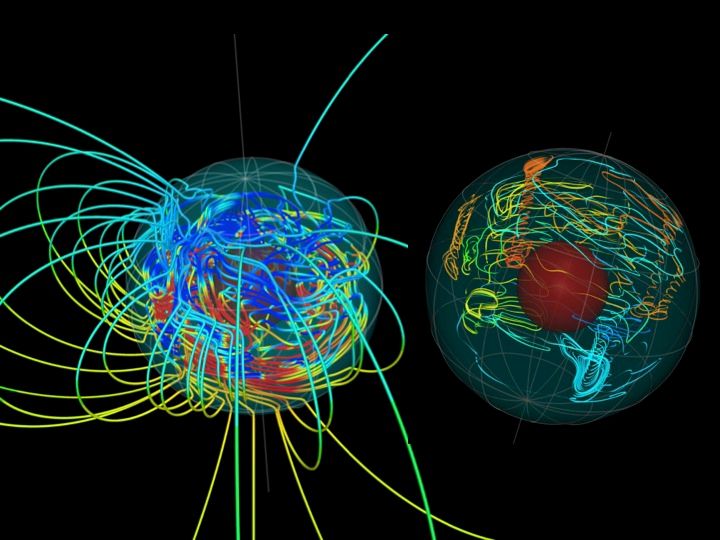
Geodynamo
Earth possesses a strong magnetic field that is originated from the Earth’s fluid outer core (the intrinsic field). This field accounts for more than 95% of the magnetic energy of the observed geomagnetic field at the Earth’s surface. Its temporal and spatial variations hold some of the key knowledge of Earth’s deep interior properties, of interactions and responses between the fluid core and the solid Earth, and of the Earth system evolution history. Therefore, understanding, interpreting and predicting geomagnetic variability are among the most active Earth research areas, and are part of NASA’s Earth science strategic objectives.
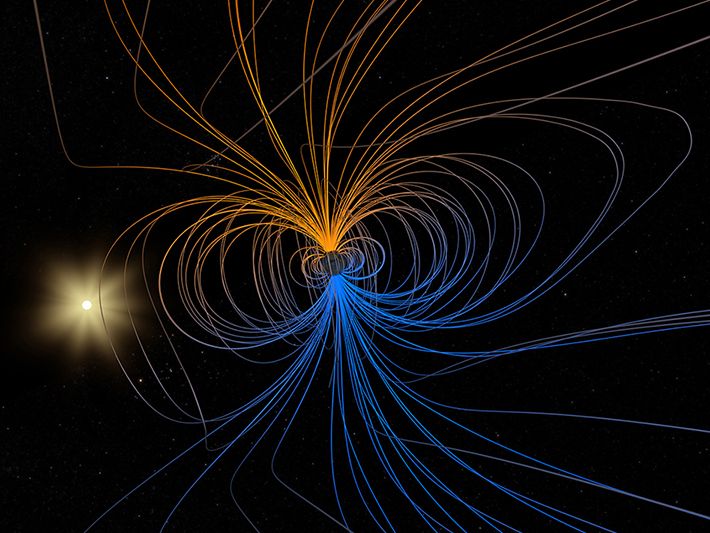
Geomagnetism
A single measurement of the near-Earth magnetic field (within 1500 km of the surface) is comprised of a superposition of signals from different current sources within the system. In order to study a particular source, it must be separated from the others using inverse theory in which the geophysical parameters of a model are inferred from field measurements.
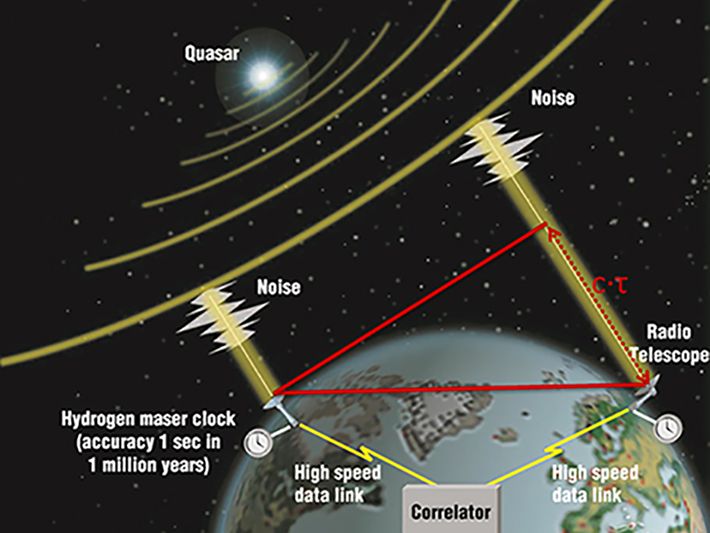
VLBI
Over its 40-year history of development and operation, the space geodetic technique called Very Long Baseline Interferometry (VLBI) has provided an unprecedented record of the motions of the solid Earth.
